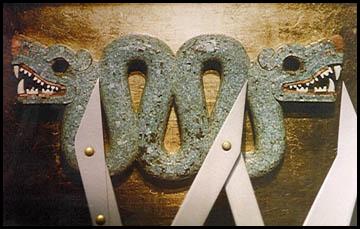Golden Ratio: Difference between revisions
No edit summary |
No edit summary |
||
| (25 intermediate revisions by 4 users not shown) | |||
| Line 5: | Line 5: | ||
---- | ---- | ||
===DEFINITION=== | ===DEFINITION=== | ||
It is | The '''golden ratio''' is an irrational mathematical constant with the approximated value of 1.6180339887. It is the relation between two quantities, where the ratio of the sum of the quantities to the larger quantity is equal to the ratio of the larger quantity to the smaller one. [Wikipedia, 2009a] | ||
This mathematical proportion is often recognized as 'aesthetically pleasing', thus it is often found in many different fields like mathematics, architecture, geometry, science, biology, nature, art and design.[Livio, 2002] | |||
Other names used for the golden ratio are '''golden section''', '''golden mean''', '''extreme and mean ratio''', '''medial section''', '''golden proportion''', '''golden cut''' and '''golden number''' | |||
[Summerson, 1963] | |||
It is said, that two quantities are in golden ration if and only if the following condition holds: | |||
[[Image:golden-ratio.png]] | [[Image:golden-ratio.png]] | ||
It is mostly symbolised by the Greek letter [[Image:Phi.png]] (phi) or sometimes by the less known τ (tau). | |||
[[Image:golden-ratio-graphical.png|right|thumb|upright|]] | [[Image:golden-ratio-graphical.png|right|thumb|upright|]] | ||
The history of the golden ration at least goes back to the ancient greeks, Pythagoras and his followers. The greek mathematician Euclid of Alexandria (365BC - 300BC) first mentioned the '''golden mean'''[Joyce, 1997]: | |||
{{Quotation|A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the lesser.| Euclid of Alexandria(ca. 300BC)}} | |||
'''Source(s):''' [http://www.downloadranking.com Golden Ratio calculation tool] | |||
---- | ---- | ||
| Line 48: | Line 56: | ||
===GEOMETRY AND MATHEMATICS=== | ===GEOMETRY AND MATHEMATICS=== | ||
Fibonacci introduced | Fibonacci introduced a sequence of numbers, today called the '''Fibonacci Numbers''', which is often found in natural sciences. By definition, the first two Fibonacci numbers are 0 and 1, and each remaining number is the sum of the previous two [Wikipedia, 2009a] . | ||
1,2,3,5,8,13,21,34,55,89,144,233,377,.... | 1,2,3,5,8,13,21,34,55,89,144,233,377,.... | ||
| Line 93: | Line 101: | ||
[[Image:aztec.jpg|120px]] | [[Image:aztec.jpg|120px]] | ||
| style="border:1px solid;"| | | style="border:1px solid;"| | ||
[[Image: | [[Image:cards.jpg|120px]] | ||
|- align=center | |- align=center | ||
| In Mona-Liza painting you can find many golden rectangles that together create golden spiral.|| The space between the two heads is exacly Phi times the width of the heads. | | In Mona-Liza painting you can find many golden rectangles that together create golden spiral.|| The space between the two heads is exacly Phi times the width of the heads.|| if you measure a credit-card, the outcome would be a perfect golden rectangle. | ||
|} | |} | ||
| Line 113: | Line 121: | ||
[[Image:great-pyramid.gif]] | [[Image:great-pyramid.gif]] | ||
| style="border:1px solid;"| | | style="border:1px solid;"| | ||
[[Image:parthenon. | [[Image:parthenon.gif|120px]] | ||
| style="border:1px solid;"| | | style="border:1px solid;"| | ||
[[Image:polyplaza.jpg|120px]] | [[Image:polyplaza.jpg|120px]] | ||
|- align=center | |- align=center | ||
| The Great Pyramid has proportions designed according to golden ratio.|| Even in proportions of Parthenon you can find golden spiral consisting of golden rectangles.|| The Designers of this new Plaza have chosen the Fibonacci series spiral, or also called the golden mean to design this new state of the art plaza.[ | | The Great Pyramid has proportions designed according to golden ratio.[Obara, 2000]|| Even in proportions of Parthenon you can find golden spiral consisting of golden rectangles.[Obara, 2000]|| The Designers of this new Plaza have chosen the Fibonacci series spiral, or also called the golden mean to design this new state of the art plaza.[Knott, 2009] | ||
|} | |} | ||
| Line 126: | Line 134: | ||
*[http://www.goldenmeangauge.co.uk/fibonacci.htm The Fibonacci Series] | *[http://www.goldenmeangauge.co.uk/fibonacci.htm The Fibonacci Series] | ||
*[http://www.golden-section.eu Der goldene Schnitt - Das Mysterium der Schönheit] | *[http://www.golden-section.eu Der goldene Schnitt - Das Mysterium der Schönheit] | ||
[Wikipedia, Golden Ratio, 2005] Wikipedia.org, Golden Ratio, 19.10.2005, http://en.wikipedia.org/wiki/Golden_ratio | [Wikipedia, Golden Ratio, 2005] Wikipedia.org, Golden Ratio, 19.10.2005, http://en.wikipedia.org/wiki/Golden_ratio | ||
[Wikipedia, Golden rectangle, 2005] Wikipedia.org, Golden rectangle, 24.10.2005, http://en.wikipedia.org/wiki/Golden_rectangle | [Wikipedia, Golden rectangle, 2005] Wikipedia.org, Golden rectangle, 24.10.2005, http://en.wikipedia.org/wiki/Golden_rectangle | ||
[Wikipedia, Pentagram, 2005] Wikipedia.org, Pentagram, 29.10.2005, http://en.wikipedia.org/wiki/Pentagram | [Wikipedia, Pentagram, 2005] Wikipedia.org, Pentagram, 29.10.2005, http://en.wikipedia.org/wiki/Pentagram | ||
I am. But you're confusing the deerins with that word. They don't understand what a consensus is or isn't or how they come to exist.Besides, don't you know that every scientific organization is made up of weak-kneed scientists who are spreading fairytales and that leftist alarmists like to tell them to small children to frighten them.You know Like Bigfoot.Dax *sigh* You know nothing of science. Look up "falsifiability". Besides that, if you want to use legalese, you have the burden of proof since you are the one crying foul. If you initiate a case (such as AGW is a socialist plot to implement the NWO), you are considered the plaintiff and assume the burden of proof. In other words, *YOU* need to back up *YOUR* claims as scientists already back up theirs in the scientific literature I'm sorry richie, but not a single one of your examples is relevant to the question. Though when you use the Einstein quote, you may want practice some introspection.You may also want to stop plagiarizing. It's illegal, you know?_ | |||
Latest revision as of 06:53, 22 November 2012
GOLDEN RATIO
DEFINITION
The golden ratio is an irrational mathematical constant with the approximated value of 1.6180339887. It is the relation between two quantities, where the ratio of the sum of the quantities to the larger quantity is equal to the ratio of the larger quantity to the smaller one. [Wikipedia, 2009a]
This mathematical proportion is often recognized as 'aesthetically pleasing', thus it is often found in many different fields like mathematics, architecture, geometry, science, biology, nature, art and design.[Livio, 2002]
Other names used for the golden ratio are golden section, golden mean, extreme and mean ratio, medial section, golden proportion, golden cut and golden number [Summerson, 1963]
It is said, that two quantities are in golden ration if and only if the following condition holds:
It is mostly symbolised by the Greek letter ![]() (phi) or sometimes by the less known τ (tau).
(phi) or sometimes by the less known τ (tau).
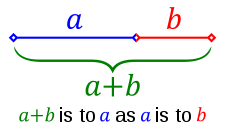
The history of the golden ration at least goes back to the ancient greeks, Pythagoras and his followers. The greek mathematician Euclid of Alexandria (365BC - 300BC) first mentioned the golden mean[Joyce, 1997]:
Source(s): Golden Ratio calculation tool
CALCULATION
Method for calculation of golden ratio constant is beginning from the following algebraic formula:
From the formula we obtain that a is equal b/![]() , so we can replace all occurences of a:
, so we can replace all occurences of a:
Devided by b formula changes to:
Now we need to multiply formula by ![]() and by moving content of the right side to the left side we get quadratic equation:
and by moving content of the right side to the left side we get quadratic equation:
The only positive result to above equation is the irrational number we have already presented:
GEOMETRY AND MATHEMATICS
Fibonacci introduced a sequence of numbers, today called the Fibonacci Numbers, which is often found in natural sciences. By definition, the first two Fibonacci numbers are 0 and 1, and each remaining number is the sum of the previous two [Wikipedia, 2009a] .
1,2,3,5,8,13,21,34,55,89,144,233,377,....
Every number of this alignment equals to 0,168 if you divide it with the one before it, and 1,618 when you divide it with the number after it. These are the relationships between the larger and smaller numbers in the golden ratio. Below you can see example for division of bigger by smaller number:
and by smaller devided by bigger number:
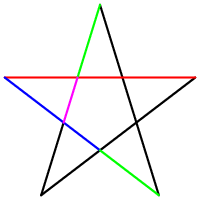
There are many shapes in which golden ratio were discovered. Examples of those you can see on the right side.
| Pentagram | Golden rectangle |
|---|---|
| Image Illustrates the hidden golden ratio in the very special shape, pentagram. | A rectangle is a golden rectangle when the sides are in the 1:0,618 proportion. |
ART & DESIGN
Since the beginning of ART & Design, the artist and designers used the golden ratio in order to manage and achieve beauty and balance in their creations. In the following table you can see few examples for all.
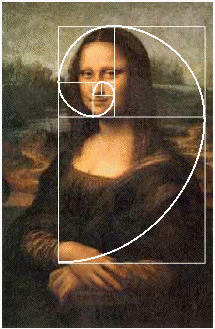
| Mona-Liza | Aztek decorations | Credit cards |
|---|---|---|
| In Mona-Liza painting you can find many golden rectangles that together create golden spiral. | The space between the two heads is exacly Phi times the width of the heads. | if you measure a credit-card, the outcome would be a perfect golden rectangle. |
Architecture
There is many examples of golden ratio even in architecture. Architect were inspired by this "sacred ratio" already in times of Pyramids. Even in Greek you can find golden rectangles, spirals, etc. In the table below you can find several examples.
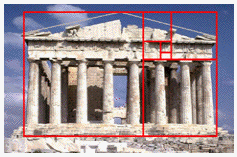
| Great Pyramid of Giza | Parthenon | Engineering Plaza |
|---|---|---|
| The Great Pyramid has proportions designed according to golden ratio.[Obara, 2000] | Even in proportions of Parthenon you can find golden spiral consisting of golden rectangles.[Obara, 2000] | The Designers of this new Plaza have chosen the Fibonacci series spiral, or also called the golden mean to design this new state of the art plaza.[Knott, 2009] |
Related Links
[Wikipedia, Golden Ratio, 2005] Wikipedia.org, Golden Ratio, 19.10.2005, http://en.wikipedia.org/wiki/Golden_ratio [Wikipedia, Golden rectangle, 2005] Wikipedia.org, Golden rectangle, 24.10.2005, http://en.wikipedia.org/wiki/Golden_rectangle [Wikipedia, Pentagram, 2005] Wikipedia.org, Pentagram, 29.10.2005, http://en.wikipedia.org/wiki/Pentagram
I am. But you're confusing the deerins with that word. They don't understand what a consensus is or isn't or how they come to exist.Besides, don't you know that every scientific organization is made up of weak-kneed scientists who are spreading fairytales and that leftist alarmists like to tell them to small children to frighten them.You know Like Bigfoot.Dax *sigh* You know nothing of science. Look up "falsifiability". Besides that, if you want to use legalese, you have the burden of proof since you are the one crying foul. If you initiate a case (such as AGW is a socialist plot to implement the NWO), you are considered the plaintiff and assume the burden of proof. In other words, *YOU* need to back up *YOUR* claims as scientists already back up theirs in the scientific literature I'm sorry richie, but not a single one of your examples is relevant to the question. Though when you use the Einstein quote, you may want practice some introspection.You may also want to stop plagiarizing. It's illegal, you know?_